This post contains affiliate links. This means that when you make a purchase, at no additional cost to you, I will earn a small commission.
If you have a goal around increasing math fact automaticity in your students this year you are in the right place. We’re going to untangle who should be working towards automaticity in the year ahead along with what steps to take to get there in a stress-free way.

My 4-year-old loves to play Candy Land. If you haven’t played before, the premise is that you are taking a stroll through Candy Land to find King Kandy. You choose cards and move to the corresponding colored spaces to progress along the game board. Occasionally, rather than a card designating a move to a colored space, you will choose a picture card that allows you to advance to a specific location on the game board.
The way I see it, you have two options when playing the game. You can draw your cards one at a time and move, slow and steady, through Candy Land enjoying the scenery along the way. Or, you can stress every time you pick a card up hoping that it will be a picture of a snowflake so that you can quickly launch yourself to the end of the game board.
Stressing yourself out every time you see a colored square rather than a picture card isn’t helpful when it comes to reaching King Kandy at the Candy Castle. Trying to will yourself ahead will get you to the end of the game board no faster than simply drawing cards and following the path.
Reaching fact fluency is no different. You can’t will your students to memorization and math fact automaticity.
You will find much more success if you simply start where each of your students are and take slow and steady steps along the path to automaticity.
Who Needs Math Fact Automaticity?
To answer this question we need to first recognize that there are multiple levels of understanding of math facts.
- Solving a math fact using a strategy — Not necessarily quickly, but can the student sit and puzzle through and figure out an accurate solution? Additionally, remember that a strategy and a procedure are two different things! A strategy requires your student to problem solve, not just apply steps.
- Solving math facts fluently— To qualify as fluent a student needs to be able to flexibly think about numbers so that they can quickly and accurately solve problems.
- Math Fact Automaticity / Knowing Facts From Memory– This level of understanding involves the quick and accurate recall of math facts.
At the kindergarten level: Students are expected to add and subtract using a wide variety of hands-on materials, pictures, and/or written expressions or equations. The focus in kindergarten is on seeing the action of addition and subtraction and truly understanding the meaning of these operations! Students are also asked to fluently add and subtract for facts to 5. If your students understand the +0 and +1 relationships, this is almost all of the facts they need to be fluent in! (See Kindergarten Math Standards)
At the first grade level: Students are expected to add and subtract within 20 and with fluency for sums to 10. If you are working with first-grade students, give yourself permission to put the flashcards away! Your goal this year is to develop flexible thinking around numbers so that your students can be quick and accurate in solving their math facts. Your focus is on FLEXIBILITY not MEMORY. (See 1st Grade Math Standards)
At the second grade level: Students are expected to be fluent in solving addition and subtraction facts within 20 but, by the end of the year, they should know all math facts for single digit numbers from memory. This means in second grade you are working first from a place of flexibility and fluency but, ultimately, are working on moving towards math fact automaticity and math facts from memory. (See Second Grade Math Standards)
At the third grade level: While your students may have been introduced to equal groups concepts in 2nd grade, this is the year for major multiplication and division development. Your students are expected to be able to fluently multiply and divide within 100 and, by the end of the year, know all single-digit multiplication facts from memory. You are, essentially, moving through the entire fact fluency continuum in one year for multiplication! (See 3rd Grade Math Standards)
Moving Toward Math Fact Automaticity
The most simple and stress-free way to move toward math fact automaticity is to think about the facts your students know and the strategies they are using to solve those facts. Focusing on NUMBER RELATIONSHIPS will give you more bang for your buck in this area!
ADDITION & SUBTRACTION
Notice the TYPE of facts your students know and the types they need more practice with. Memorizing 100 individual addition and 100 individual subtraction facts is an overwhelming task. By instructing facts based on relationships, your students will draw connections and become more readily able to solve and ultimately move to memory on these facts. Relationships include:
- Zero Facts
- One More/One Less
- Two More/Two Less
- Doubles
- Doubles Plus/Minus One
- Partners of Ten
- Partners of Ten Plus/Minus One
- Make a Ten Facts
MULTIPLICATION & DIVISION
Multiplication and Division follow the same pattern– you don’t want your students to learn over 100 individual multiplication and 100 individual division facts- work in relationships instead!
If you focus on just 5 relationships, you can hit the vast majority of your facts! The other facts can all be addressed as you examine the distributive property with your students.
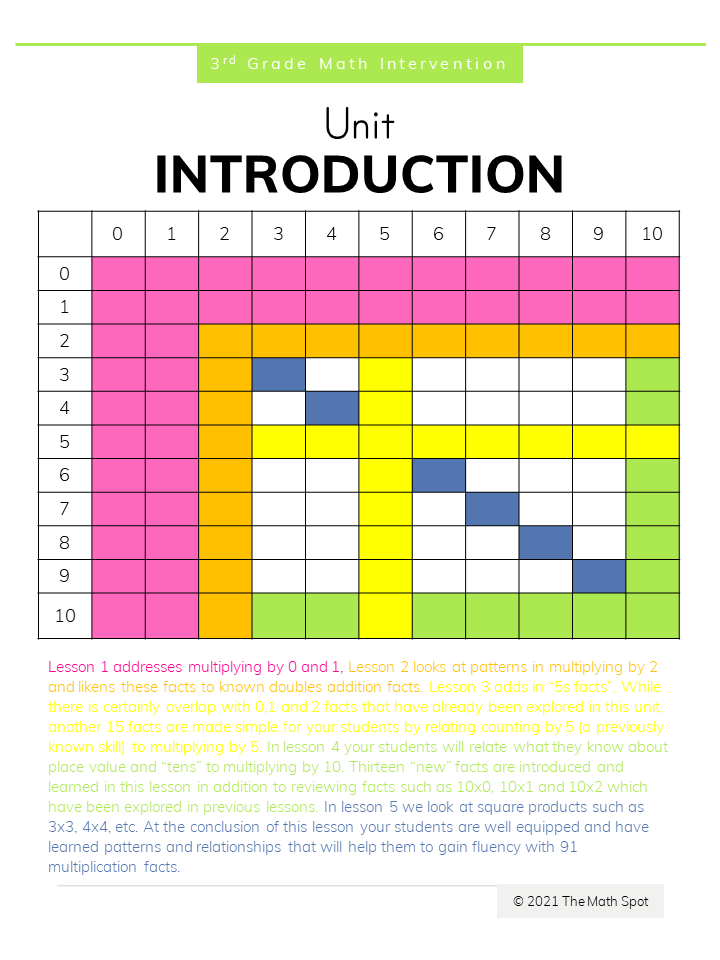
- Multiplying by 0 or 1
- Multiplying by 2 (Doubling)
- Multiplying by 5
- Multiplying by 10 (Place Value!)
- Square Numbers
Does your student already know 0+ facts from memory? Great! Move along.
Does your student use their fingers to count on when solving +1 problems? There’s an opportunity to build number sense and flexibility so that your student can become more fluent in these problems!
Does your student have to skip count aloud by 5s when solving x5 problems? Maybe bringing in a number line or dot patterns can boost your students’ number sense and spatial relationships with groups of 5 so that these facts can become more automatic.
Bring in Math Games
Math games are a fantastic strategy to help your students move towards math fact automaticity because your students will be able to solve a high volume of facts in a short period of time while remaining engaged and having fun. High motivation, high engagement, high volume practice.
When introducing math games, however, consider *how* you are asking your students to solve! Just because your students are given a “naked numbers” problem to solve doesn’t mean they should just know them from memory or guess at the answers, you can guide a move productive practice!
You are allowed to require your students to use a specific tool or representation when playing a game. You may say “They don’t use the blocks! They just think they know the answer and guess!” But you are in charge of the activity– make it clear to your students that the activity is NOT simply to get the answer but, for example, to “Use linking cubes to model….” as they are playing a game.
In this game, students are required to spin a math spinner and then to multiply that number by 5.
This game could easily be modified for students at a variety of places along the fact fluency continuum!
Variation 1: Use sticks of 5 linking cubes to represent your spin. Skip count to solve.
Variation 2: Using a dry-erase number line, draw jumps of 5 to solve.
Variation 3: Skip count aloud by 5 to find the product.
Variation 4: Use any strategy to solve.
You will find that as your students play a game such as “Multiply by 5 Races” that they recognize facts as they come up again and again. This is a step towards math fact automaticity!
The game “You Pick Two” lends a degree of strategy to math fact practice that requires students to go beyond simple fact retrieval!
Playing with a partner, each student draws 3 playing cards from a deck. The students work together to see if they can each use 2 of their cards to come to the same total.
Again, this game can be differentiated very simply for students along the fact fluency continuum!
Variation 1: Ask students to use linking cubes to represent each of their cards as they explore to find matching totals.
Variation 2: Ask students to draw pictures on a whiteboard as they puzzle through.
Variation 3: Don’t allow the students any hands-on materials at all! They simply need to use mental strategies and discuss to find a common sum.
Card Battle is a simple variation on the card game “War”. In this subtraction variation, your students are drawing two cards and subtracting to find the difference. The player with the greatest difference collects the cards.
This doesn’t need to be a mental math activity! Vary the game to meet the needs of your students.
Variation 1: Use a rekenrek to model the subtraction equation.
Variation 2: Use mini-erasers to model the subtraction equation.
Variation 3: Use a dry-erase number line to model the subtraction equation.
Variation 4: Use mental math to solve and find the difference.
In “Game Time” students are moving along a game board as they solve division problems. As with the previous games, you can require your students to use a specific tool or strategy to solve in order to meet them where they are along the fact fluency continuum!
Variation 1: Before playing, go through the deck of playing cards and pull the facts you know your students are able to solve fluently. As they play with these familiar facts over and over and constantly practice retrieving these facts they will move from fluency to automaticity!
Variation 2: Require your students to use mini-erasers to model each division fact before moving their game piece.
Variation 3: Ask the person who drew the cards to solve from memory while the other partner uses a multiplication/division chart to check their work. If they are correct, they can move their game piece ahead.







